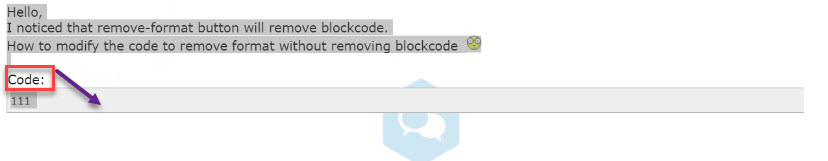
I noticed that remove-format button removes blockcodes.
Is it possible to preserve blockcodes (as it preserves blockquotes) by modifying code of SCEditor

I have another question about remove-format function:
The font-size cannot be cleared in one click if it is nested (this is common in web page HTML)
For example, I copied some paragraphs from wikipedia
- Code:
[size=14]In[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Physics][size=14]physics[/size][/url][size=14],[/size][size=14] [/size][b][size=14]angular momentum[/size][/b][size=14] [/size][size=14](rarely,[/size][size=14] [/size][b][size=14]moment of momentum[/size][/b][size=14] [/size][size=14]or[/size][size=14] [/size][b][size=14]rotational momentum[/size][/b][size=14]) is the rotational equivalent of[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Linear_momentum][size=14]linear momentum[/size][/url][size=14]. It is an important quantity in physics because it is a[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Conservation_law][size=14]conserved quantity[/size][/url][size=14]—the total angular momentum of a closed system remains constant.[/size]
[size=14]In three [url=https://en.wikipedia.org/wiki/Dimensions]dimensions[/url], the angular momentum for a [url=https://en.wikipedia.org/wiki/Point_particle]point particle[/url] is a [url=https://en.wikipedia.org/wiki/Pseudovector]pseudovector[/url] [b]r[/b] × [b]p[/b], the [url=https://en.wikipedia.org/wiki/Cross_product]cross product[/url] of the particle's [url=https://en.wikipedia.org/wiki/Position_vector]position vector[/url] [b]r[/b] (relative to some origin) and its [url=https://en.wikipedia.org/wiki/Momentum_vector]momentum vector[/url]; the latter is [b]p[/b] = [i]m[/i][b]v[/b] in Newtonian mechanics. Unlike momentum, angular momentum depends on where the origin is chosen, since the particle's position is measured from it.[/size]
[size=14]Just as for [url=https://en.wikipedia.org/wiki/Angular_velocity]angular velocity[/url], there are two special types of angular momentum of an object: the [i]spin angular momentum[/i] is the angular momentum about the object's [url=https://en.wikipedia.org/wiki/Centre_of_mass]centre of mass[/url], while the [i]orbital angular momentum[/i] is the angular momentum about a chosen center of rotation. The total angular momentum is the sum of the spin and orbital angular momenta. The orbital angular momentum vector of a point particle is always parallel and directly proportional to its orbital [url=https://en.wikipedia.org/wiki/Angular_velocity]angular velocity[/url] vector [b]ω[/b], where the constant of proportionality depends on both the mass of the particle and its distance from origin. The spin angular momentum vector of a rigid body is proportional but not always parallel to the spin angular velocity vector [b]Ω[/b], making the constant of proportionality a second-rank [url=https://en.wikipedia.org/wiki/Tensor]tensor[/url] rather than a scalar.[/size]
[size=14]Angular momentum is an extensive quantity; i.e. the total angular momentum of any composite system is the sum of the angular momenta of its constituent parts. For a [url=https://en.wikipedia.org/wiki/Continuum_mechanics]continuous[/url] rigid body or a fluid the total angular momentum is the volume integral of angular momentum density (i.e. angular momentum per unit volume in the limit as volume shrinks to zero) over the entire body.[/size]
[size=14]Torque can be defined as the rate of change of angular momentum, analogous to [url=https://en.wikipedia.org/wiki/Force]force[/url]. The net [i]external[/i] torque on any system is always equal to the [i]total[/i] torque on the system; in other words, the sum of all internal torques of any system is always 0 (this is the rotational analogue of [url=https://en.wikipedia.org/wiki/Newton%27s_Third_Law]Newton's Third Law[/url]). Therefore, for a [i]closed[/i] system (where there is no net external torque), the [i]total[/i] torque on the system must be 0, which means that the total angular momentum of the system is constant. The conservation of angular momentum helps explain many observed phenomena, for example the increase in rotational speed of a spinning [url=https://en.wikipedia.org/wiki/Figure_skater]figure skater[/url] as the skater's arms are contracted, the high rotational rates of [url=https://en.wikipedia.org/wiki/Neutron_star]neutron stars[/url], the [url=https://en.wikipedia.org/wiki/Coriolis_effect]Coriolis effect[/url], and the [url=https://en.wikipedia.org/wiki/Precession]precession[/url] of [url=https://en.wikipedia.org/wiki/Gyroscope]gyroscopes[/url]. In general, conservation limits the possible motion of a system but does not uniquely determine it.[/size]
[size=14]In[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Quantum_mechanics][size=14]quantum mechanics[/size][/url][size=14], angular momentum (like other quantities) is expressed as an[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Operator_(physics)][size=14]operator[/size][/url][size=14], and its one-dimensional projections have[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Point_spectrum][size=14]quantized eigenvalues[/size][/url][size=14]. Angular momentum is subject to the[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Heisenberg_uncertainty_principle][size=14]Heisenberg uncertainty principle[/size][/url][size=14], implying that at any time, only one[/size][size=14] [/size][url=https://en.wikipedia.org/wiki/Vector_projection][size=14]projection[/size][/url][size=14] [/size][size=14](also called "component") can be measured with definite precision; the other two then remain uncertain. Because of this, the axis of rotation of a quantum particle is undefined. Quantum particles[/size][size=14] [/size][i][size=14]do[/size][/i][size=14] [/size][size=14]possess a type of non-orbital angular momentum called "spin", but this angular momentum does not correspond to a spinning motion.[/size][sup][size=12][url=https://en.wikipedia.org/wiki/Angular_momentum#cite_note-1][1][/url][/size][/sup]
After first click, there are 28 pairs left
After second click, there are 0 pairs left
Is it possible to clear all font-size in one click



 by hbghlyj August 20th 2021, 11:00 am
by hbghlyj August 20th 2021, 11:00 am